Continuities of the Discontinuous: Mathematics and Humanism in Mid-century Germany

This is part of our Campus Spotlight on Princeton University.

 

In 1913, the Austrian writer Robert Musil made an intriguing if not unsettling observation: among the mathematically inclined, there appeared to be two, diametrically opposed ideas about the relationship of mathematics to the “real world.” Scientists, engineers, and technocrats, Musil noted, exhibited great alacrity in adapting mathematical equations to serve as the foundation for a lived world growing ever more technical and quantified. At the same time, actual mathematicians—the theorists puzzling over arcane and indecipherable chalkboards—were broaching a reality of their discipline that still obtains today: “something in the foundations of the entire thing,” Musil notes, “was totally unreconcilable; they dug down to the very bottom of it all and ended up discovering that: there was no foundation, no bottom on which everything in fact stood.”[1] While many readers may be nonplussed that no one bothered to inform their calculus instructors that the totality of mathematics is theoretically unjustifiable, others may also note that this idea has in no way hindered the degree to which everything from our love lives to our sleep patterns has been subject to a steady creep of digitization and quantification. But Musil nevertheless put his finger on a potentially enormous paradox. If the foundational crisis (die Grundlagenkrise) with which mathematicians had been wrestling since the mid-1800s indicated that mathematics did not make sense mathematically, should this not worry a society whose own foundations—be they physical, political, or economic—rested so heavily on numericity?

In the twentieth-century Germanophone world, this crisis of mathematical thought came to be viewed as symptomatic of a broader crisis of rationality that found its most horrific expression in the fascist catastrophe and the systematic murder of the Holocaust. Already in the decades leading up to the war, philosopher Edmund Husserl articulated a deep suspicion of the intellectual tradition in which nature is understood as a mere “mathematical manifold,” and he rejected any equivocation between mathematical rationality and lived experience. After the war, the chorus of voices echoing Husserl’s position only grew. Perhaps the most canonical of these anti-mathematical arguments belong to the so-called Frankfurt School. Theodor W. Adorno, Max Horkheimer, Herbert Marcuse, and Jürgen Habermas all attacked mathematical rationality for what they perceived to be its reductive approach to the world, its constraining and conforming logics, and a naïve positivism that was all too cozy with capitalist hegemony. In their Dialectic of Enlightenment, Adorno and Horkheimer explicitly rejected mathematical thinking as merely another form of mythology, another of the myriad errors that keep society shackled to barbarism. As the Frankfurt School’s critique of fascism grew to represent a canonical position of postwar, progressive intellectualism, its deep distrust of mathematical and, by extension, technological thought established itself among many anti-fascist thinkers as a natural, even necessary stance for a critical approach to society. But Musil’s observations reveal a paradoxical resilience within mathematical thought: even in the depths of the discipline’s foundational crisis, mathematicians continued to advance new theoretical claims, and, more bafflingly perhaps, the world continued to rely on mathematical logics with an apparently ever-growing stolidity.[2] To the extent that mathematics is marked by a conceptual resiliency in the face of crisis, should there not also exist intellectuals who turned to mathematical thought for a model of tenacious perseverance and continuity within discontinuity that might aid in rebuilding the ruins left by German fascism?

My research poses just this question. It reexamines the intellectual genealogies of the past century, unearthing a lineage of theoretical positions that approach mathematics as one foundation of rational humanisms, anti-fascist politics, and utopian technocracies. Indeed, throughout the twentieth century, a significant number of German-language intellectuals advanced anti-fascist, humanist arguments that deployed mathematics, technology, and rationalism as their guiding conceits.[3] For many of these figures, the question of the human had become (or had always been) inseparable from mathematical rationality and its application in the material world. These positions are of a great significance because they both prefigure and critically address our own moment of algorithmic saturation. Understanding the justifications of this mathematical and rational humanism sheds lights on one intellectual path leading to the present day, but it also holds the potential of revealing overlooked warnings and past alternatives to the social, political, and interpersonal crises of the digital age. My dissertation, titled “Mathematik ist immer Geist: The Persistence of Mathematical Humanism and Aesthetic Rationality in Postwar Germany,” maps this largely disregarded intellectual ecology.[4]

This project undertakes close analysis of three case studies. The first represents perhaps the most stalwart advocate in the German postwar for a mathematical humanism or, in his words, “existential rationalism,” the polymath Max Bense (1910–1990). This study follows Bense’s interest in “encyclopedism”—derived from the historical Encyclopédie of Denis Diderot and Jean le Rond d’Alembert—as a liberal, social, and democratic model that might serve as a corrective to an otherwise bankrupt Enlightenment tradition. My research reveals in Bense’s early work the mathematical intellectual history that informs the radical epistemological pluralism he identifies as a hallmark of encyclopedic thinking. This “mathematicity” brings with it a politics of intelligence, creating unexpected links between the encyclopédistes, mathematical set theory from the late-nineteenth century, and an optimism for a future, humanist technocracy. At intellectually crucial moments, Bense invokes encyclopedism a) to understand the world inhabited (and exclusively inhabitable) by humans as a fundamentally material and mechanical phenomenon; b) as a method for endowing mathematics with not just an essentially technical character, but one that is thereby also human-centered; and c) as an intellectual conceit that promotes epistemologies that are at heart plural, open, and always processual, that is, never complete or totalizing. Encyclopedism unites Bense’s ongoing interests in mathematics, technics, and knowledge production, priming what will become his embrace of cybernetics, information theory, and semiotics beginning in the 1950s, and it forms a crucial justification for the anti-fascist politics of intelligence that he proposes in the war’s aftermath. Equally the subject of his theoretical focus as a description of his work’s character, Bense’s mathematically inflected encyclopedism represents a distinct conceptual and moral stance in a thoroughly and permanently technical world.

Turning to questions of consumer society, the second case study examines the Hochschule für Gestaltung (or HfG; 1953–1968) in the West German city of Ulm, a post-Bauhaus design school where a conviction about the quantifiability of the world is bound up with an aim to create, all at once, a more efficient, more critical, and more just mode of living. At stake is the fundamentality of the “unit” in Ulm design ideology, both in its necessary legibility as a discrete element and its fundamental combinability with other units. For Ulmers, the singular, divisible, discrete unit is not only rooted in a particular mathematical sensibility, but also—perhaps surprisingly—explicitly linked to an optimism about a consumer economy unchained from profit and surplus. My study tracks this combinatoric design ethos from its most abstract expressions in the reimagining of architectural space as polyhedral matrices, to its most mundane manifestations in Ulm’s emphasis on stackable and interlocking product packaging. At Ulm, combinatorics is even present as a major influence on developments in aesthetic theory and experimental poetic forms. Foremost is the discretization of poetic elements, the (re)combination of these poetic units, and the importance of symmetrical arrangements in early German-language Concrete poetry, whose representative, Eugen Gomringer, was a central figure in 1950s Ulm. Emerging across these discussions is the conceptual importance—here implicit, there explicit—of group- and set-theoretical mathematics, forms of analysis that emerged from the Grundlagenkrise and are concerned with numeration, orderings, and symmetries. This chapter argues not only that these mathematical principles are integral to the aesthetic and conceptual interventions occurring in the context of the HfG; it also links such mathematical dispositions to the social and political stakes of a consumer position defined by globalizing networks and the ubiquity of the market.

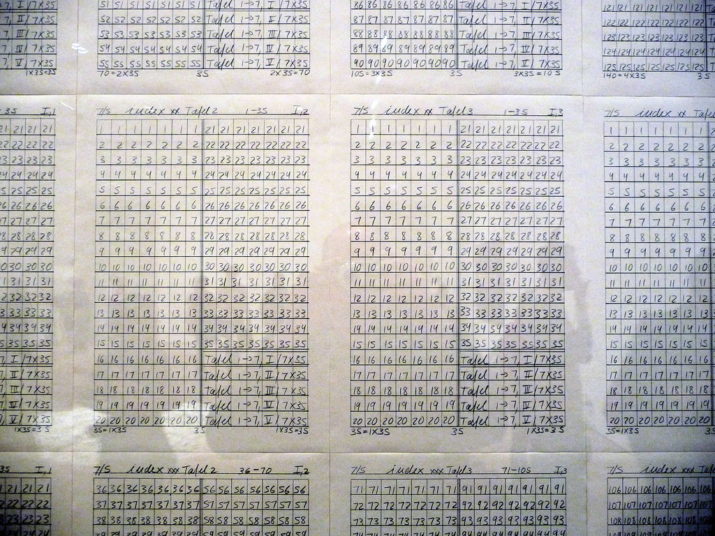
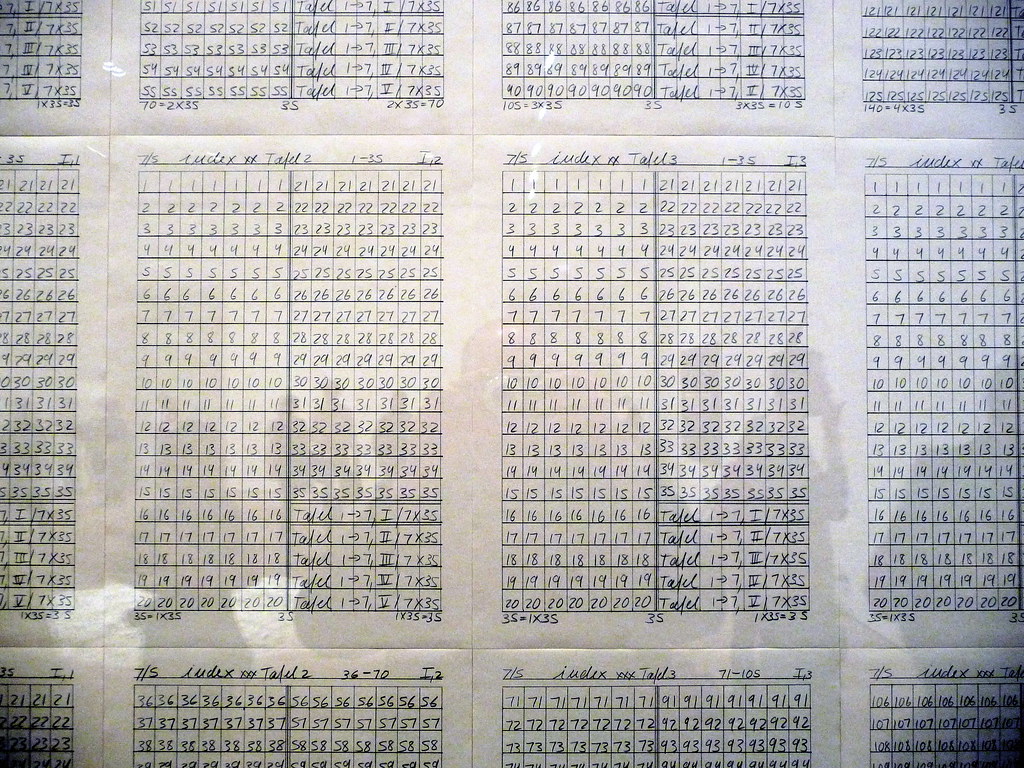
The project’s final study considers how mathematical consciousness manifests in visual art by analyzing the oeuvre of Hanne Darboven (1941–2009), an artist whose work straddles the boundaries between Conceptualism, Minimalism, and Media Art. The chapter hones in on Darboven’s interest in the Russian Constructivist El Lissitzky during the late 1960s and early 1970s, focusing specifically on the ways that Lissitzky’s own deployment of mathematical thinking inspired a revamping of the aesthetic practice that Darboven calls “mathematical prose”—an artistic mode as much about the inscription of letters and numerals as about the depiction of images or ideas. Lisstizky’s writings provide a more refined vocabulary for many of the themes incorporated into Darboven’s art, aligning aesthetics with mathematical concepts of the irrational, the imaginary, and the infinite, and casting her artworks as inherently constitutive of systems that include both the human and the extra-human. Armed with Lissitzky’s mathematically attuned aesthetic framework, the analysis then turns to Schreibzeit (literally: “time for writing”), an artwork Darboven produced primarily between 1975 and 1981. Using Lissitzky as a lens through which to interpret Schreibzeit, the chapter reveals yet unobserved principles of organization that center on part/whole relations, aphoristic collections, and encyclopedic mechanics. Key in this analysis are a number of sources cited in Schreibzeit, including the cyclically resonant Tao Te Ching, the self-referential Brockhaus Encyclopedia, and the Grundlagenkrise-treatise by Richard Dedekind, What Are Numbers and What Should They Be? It is Dedekind’s particular theory of the natural numbers as a self-referential, self-producing set that spurs the concluding conjecture that Schreibzeit advances an autopoietic aesthetic: here, the artist’s subjectivity—as site of cognition, creation, and curation—is distributed across a network of self-reflexive operations that constitute the work as they describe it, recursively generating the artwork to which they already belong. In Darboven’s work, mathematical consciousness thus even serves as an engine beyond humanism and toward the development of a distributed, posthuman mode of expression.

Taken together, the chapters of my dissertation show how mathematical thought came to bear on the renegotiation of the social fabric in capitalist West Germany, and on the aesthetic forms that sought to capture and critique that fabric. The cases I trace approach mathematical rationality, industrial technology, and environmental systems as unavoidable conditions structuring lived reality, but seek ways to harness that mathematical and technological rationalism in support of a liberal, democratic collective as well as new aesthetic and literary modes. While the figures I examine all advocate for critical approaches to rationalist regimes, they also all acknowledge those regimes’ radical contingency—humans made them, thus humans can alter them—and tender a cautious optimism toward their potential for more equitable, more humane modes of living. My case studies thus explore the dictum of Bense’s that I borrow for my title: “Mathematik ist immer Geist” (for a note on translation, see Footnote 4). Bense’s formulation not only links mathematical thought to a complex of ideas about the philosophy of knowledge, intellectual inspiration, and cultural paradigms; it also points to the inescapable implications that mathematical thinking has for the human.

Inasmuch as this project illustrates the myriad ways that mathematics both challenged and aided a postwar humanist project, it is also about understanding how certain German-speaking intellectuals and cultural producers looked to the history of mathematics for inspiration in the face of the many crises that culminated in 1945. A century earlier, mathematics had undergone an epistemological rupture that had shaken it to its core, and yet managed to steady itself through novel reconceptualizations of foundational ideas like number, operation, and function; what might mathematics’ perseverance and constructive reorientation teach a society similarly shaken and broken? Thus, this project surveys both the depths at which mathematical thinking is embedded in German (and by extension, Western) society, and how mathematics—a discipline that is, as Musil indicates, multiply “post-crisis”—might model constructive paths forward for society brought to the brink. Above all, this research helps reveal how our current moment of technological saturation nevertheless dialogues with a legacy of anti-fascist intellectual projects. It thereby aims to recover a broader and more nuanced understanding of the critical potential in mathematical rationality for artistic expression and the lived world. This project offers crucial insight into the way that mathematical humanism marked a particularly German postwar intellectual optimism, and still bears critical lessons for the digital natives, nomads, and networks on which so much of our present moment depends.

 

William Stewart is currently a College Fellow in Harvard University’s Department of Germanic Languages and Literatures. He received a PhD jointly from the Department of German and the Interdisciplinary Doctoral Program in the Humanities at Princeton University. His writing has appeared in Grey Room, Zeitschrift für Medien- und Kulturforschung (ZMK), and the Los Angeles Review of Books.

 

References

[1] Robert Musil, “Der mathematische Mensch,” in Gesammelte Werke: Prosa und Stücke, ed. Adolf Frisé (Reinbek bei Hamburg: Rowohlt, 1978), 1006. Translation mine.

[2] This one idea is the subject of the dissertation’s first chapter: that the various crises of discontinuity within the Grundlagenkrise are reflected not only in the mathematics (i.e. the problem of the discontinuous), but also in the mathematicians’ very attitude toward the history of their own discipline. Indeed, the foundational crisis that takes place in late nineteenth-century mathematics is but one episode in a much longer tradition of rupture, reinvention, and repossession that characterizes so much of the history of mathematics. Crises in mathematics—of which there are so many—effect not so much breaks as reorientations around constantly renegotiated conceptual regimes. The history of mathematics is as much a history of intellectual “development” as it is one of hibernations, retreats, and rear-guard actions.

[3] Importantly, the perspective on the years 1933-1945 held by my subjects did not conflate fascism wholly with the murder perpetrated in the context of the Holocaust. This is an historical accident: for at least the first fifteen years following the war’s end, the imagination of the Holocaust that dominated public discourse in Germany (and Europe at large) was vague, a-semiticized, and only one atrocity among many committed by the Nazis. For many of my subjects, “fascism” is understood primarily as a form of political-intellectual control that was the essential cause of a physically destroyed German nation. Only my final case study, Hanne Darboven, exists in a period in which public discussions about the extent of the Holocaust and its essential relation to the Nazi project grew more fully fledged. For an overview of this (morally fraught) history, see Tony Judt, Postwar: A History of Europe since 1945 (New York: Penguin Press, 2005), 808ff.

[4] The German phrase before the colon is taken from Max Bense’s 1951 “Manifest des existentiellen Rationalismus” (Manifesto for an existential rationalism) and is best translated as “mathematics is always tantamount to human intellect.” Geist is a favored concept of Bense’s and equally challenging to translate. The overdetermination by the long philosophical, theological, and metaphysical traditions burdening the term is well known. In Bense’s case, several related words can adequately render his sense of Geist, including “mind/mental,” “theory/theoretical,” and “concept/conceptual.” Chief among these, however, are “intellect” and “intellectual,” as Bense contends that it is Geist that ultimately underlies society’s Intelligenz. In Bense’s case, the connotation of Geist is always rational, scientific, and mathematical. See here Max Bense, Geist der Mathematik: Abschnitte aus der Philosophie der Arithmetik und Geometrie(Munich: Richard Oldenbourg, 1939); Bense, Technische Existenz: Essays (Stuttgart: Deutsche Verlags-Anstalt, 1949), 85; and Bense, “Manifest des existentiellen Rationalismus [1951],” in Ausgewählte Schriften in vier Bänden: Band 1, Philosophie, ed. Elisabeth Walther, vol. 1 (Stuttgart: J.B. Metzler, 1998), §17.

 

 

Photo: “Seven Panels and Index,” Hanne Darboven, 1973